Plane Crash Problem - How Long Does It Take to Fall?
Free Fall of objects
Inspired by a conversation in Shenzhen, I am trying to compare the terminal velocity of a human and a plane under turbulent flow:
Consider \(a=\dot{v(t)}\), we have \(F=m\dot{v}\). Now consider downwards as positive. Assume air resistance is \(kv^2\), where \(k \in \mathbb{R}\). Then we have:
\[\begin{aligned} m\dot{v}&=mg-kv^2 \\ m \frac{dv}{dt}&=mg-kv^2 \\ mdv&=(mg-kv^2)dt \\ \frac{mdv}{mg-kv^2}&=dt \\ \int_{0}^{v} \frac{mdv}{mg-kv^2} &= \int_{0}^{t}dt \\ t&=\int_{0}^{v} \frac{mdv}{mg-kv^2} \end{aligned}\]Where we assumed \(v(0)=0\)
\[\begin{equation} \int \frac{d v}{m g-k v^2} =\frac{1}{m g} \int\frac{d v}{1-\frac{k}{m g} v^2} \\ \end{equation}\]Evaluate the indefinite integral first, if we assume \(u=\sqrt\frac{k}{mg} v\), hence \(\sqrt\frac{mg}{k} du=dv\), we have:
\[\begin{aligned} &\sqrt{\frac{m}{gk}} \int \frac{d u}{1-u^2} \\ & = \sqrt{\frac{m}{gk}} \tanh ^{-1}(u) \\ &= \sqrt{\frac{m}{gk}} \tanh ^{-1}\left(v \sqrt{\frac{k}{m g}}\right) \end{aligned}\]The reader can easily check that the definite integral is the same, i.e.
\[t= \sqrt{\frac{m}{gk}} \tanh ^{-1}\left(v \sqrt{\frac{k}{m g}}\right)\]After this we can do some algebraic manipulations to get : \begin{equation} v=\sqrt {\frac{mg}{k}}\tanh \left( t \sqrt \frac{gk}{m} \right) \end{equation}
As \(\tanh(x)\) approaches \(1\), \(v_\text{Terminal}=\sqrt{\frac{mg}{k}}\)
Next, we have to find the value of \(k\): Using drag equation, assuming turbulent flow:
\(F_{\mathrm{d}}=\frac{1}{2} \rho c_{\mathrm{d}} A v^2\) Hence:
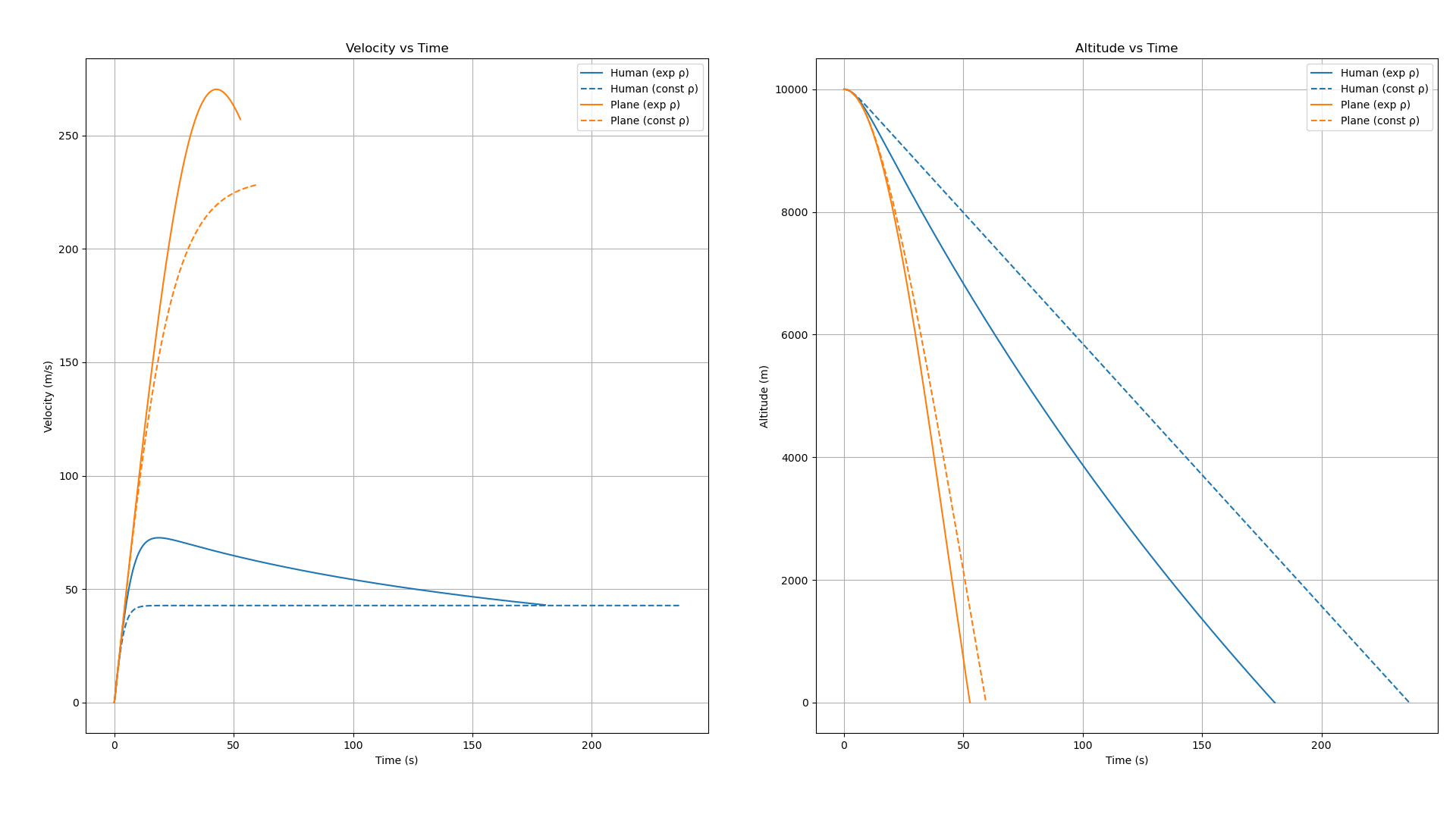
\[\begin{aligned} v_\text{Terminal}&=\sqrt{\frac{mg}{k}} \\ &=\sqrt{\frac{2mg}{\rho c_d A}} \end{aligned}\]As \(m_\text{human}\)«<\(m_\text{plane}\), the difference in \(c_d\) and \(A\) can’t compensate, and hence terminal velocity of planes are much higher during free fall.
Free Fall with Altitude-Varying Air Density
Inspired by our constant-density derivation, we now allow the air density to fall off exponentially with altitude:
\[\rho(h)=\rho_0\,e^{-h/H},\]and seek a closed-form expression for the descent speed \(v\) as a function of the instantaneous altitude \(h\).
Consider downward as positive. The net force on a body of mass \(m\) is gravity minus quadratic drag:
\[\begin{aligned} m\dot{v}&=m\,g \;-\;\tfrac12\,\rho(h)\,c_d\,A\,v^2 =m\,g \;-\;k\,e^{-h/H}\,v^2,\\ m\frac{dv}{dt}&=m\,g - k\,e^{-h/H}\,v^2, \qquad \frac{dh}{dt}=-v, \end{aligned}\]where
\[k=\tfrac12\,\rho_0\,c_d\,A.\]1. Change of Independent Variable
We trade the time derivative for an \(h\)-derivative via
\[\frac{dv}{dt} =\frac{dv}{dh}\,\frac{dh}{dt} =-\,v\,\frac{dv}{dh}.\]Set \(u(h)=v(h)^2\). Then
\[-\,v\,\frac{dv}{dh} =g - \frac{k}{m}\,e^{-h/H}\,v^2 \;\Longrightarrow\; \frac{d}{dh}\bigl(v^2\bigr) =\frac{2k}{m}\,e^{-h/H}\,v^2 - 2\,g.\]Hence
\[\frac{du}{dh} -\frac{2k}{m}\,e^{-h/H}\,u =-2\,g.\]2. Integrating Factor
The integrating factor is
\[\mu(h) =\exp\!\Bigl(-2\,\frac{k}{m}\int e^{-h/H}\,dh\Bigr) =\exp\!\bigl(2\,\tfrac{k\,H}{m}\,e^{-h/H}\bigr).\]Multiply the ODE by \(\mu(h)\) and integrate from the initial altitude \(h_0\) (where \(v(h_0)=0\implies u(h_0)=0\)):
\[\frac{d}{dh}\!\bigl(u\,\mu\bigr) =-2\,g\,\mu(h) \;\Longrightarrow\; u(h)\,\mu(h) =-2\,g \int_{h_0}^{\,h}\mu(s)\,ds.\]Since
\[\int e^{2\frac{kH}{m}e^{-s/H}}\,ds = -H\,\operatorname{Ei}\!\bigl(2\frac{kH}{m}e^{-s/H}\bigr) + C\]we obtain
\[v(h)^2 = \exp \! \left(-2\,\tfrac{kH}{m}e^{-h/H}\right)\, 2gH\left[\operatorname{Ei} \! \left(2\,\tfrac{kH}{m}e^{-h/H} \right) - \operatorname{Ei} \! \left(2\, \tfrac{kH}{m}e^{-h_0/H}\right) \right].\]3. Closed-Form Speed \(v(h)\)
Taking the square‐root yields:
\[\boxed{ v(h) =\sqrt{2\,g\,H}\; \exp\!\Bigl(-\tfrac{k\,H}{m}e^{-h/H}\Bigr)\; \sqrt{ \operatorname{Ei}\!\bigl(2\,\tfrac{k\,H}{m}e^{-h/H}\bigr) -\operatorname{Ei} \!\bigl(2\,\tfrac{k\,H}{m}e^{-h_0/H}\bigr) } }.\]4. Time as a Function of Altitude
To recover \(t(h)\), integrate
\[t(h) =\int_{h_0}^{\,h}\frac{dh'}{-\,v(h')} =\int_{h}^{\,h_0}\frac{dh'}{v(h')}.\]With the substitution
\[x=2\,\frac{k\,H}{m}\,e^{-h'/H}, \quad dh'=-\frac{H}{x}\,dx,\]one finds
\[\boxed{ t(h) =\sqrt{\frac{H}{2\,g}} \;\Bigl[ \Gamma\!\bigl(\tfrac12,\,2\,\tfrac{k\,H}{m}e^{-h/H}\bigr) -\Gamma\!\bigl(\tfrac12,\,2\,\tfrac{k\,H}{m}e^{-h_0/H}\bigr) \Bigr] },\]where \(\Gamma(s,x)\) is the upper incomplete gamma‐function.
6. Recovery of the “tanh” Solution in the Limit (H\to\infty)
We start from our closed-form time–vs–altitude relation
\[t(h) =\sqrt{\frac{H}{2g}} \Bigl[ \Gamma\!\bigl(\tfrac12,\,2\frac{kH}{m}e^{-h/H}\bigr) \;-\;\Gamma\!\bigl(\tfrac12,\,2\frac{kH}{m}e^{-h_0/H}\bigr) \Bigr].\]Next, use the gamma–error‐function identity
\[\Gamma\!\bigl(\tfrac12,\,z\bigr) =\sqrt{\pi}\,\operatorname{erfc}(\sqrt{z}).\]Define
\[y \;=\; 2\frac{kH}{m}\,e^{-h/H}, \qquad y_0 \;=\; 2\frac{kH}{m}\,e^{-h_0/H}.\]Then
\[t(h) =\sqrt{\frac{H}{2g}}\;\sqrt{\pi} \bigl[\operatorname{erfc}(\sqrt{y}) - \operatorname{erfc}\sqrt{y_0})\bigr].\]As \(H\to\infty\), both \(y,y_0\to\infty\) but their difference
\[y - y_0 =2\frac{kH}{m}\bigl(e^{-h/H}-e^{-h_0/H}\bigr) \;\longrightarrow\; 2\frac{k}{m}(h_0 - h)\]remains finite. For large argument \(w\gg1\),
\[\operatorname{erfc}(w)\sim \frac{e^{-w^2}}{\sqrt{\pi}\,w},\]so
\[\operatorname{erfc}(\sqrt{y})\approx\frac{e^{-y}}{\sqrt{\pi\,y}}, \quad \operatorname{erfc}(\sqrt{y_0})\approx\frac{e^{-y_0}}{\sqrt{\pi\,y_0}}.\]Substitute into (t(h)):
\[\begin{aligned} t(h) &\approx \sqrt{\frac{H}{2g}}\;\sqrt{\pi} \Bigl[\frac{e^{-y}}{\sqrt{\pi\,y}} -\frac{e^{-y_0}}{\sqrt{\pi\,y_0}}\Bigr] \\[6pt] &= \sqrt{\frac{H}{2g}} \Bigl[\frac{e^{-y}}{\sqrt{y}} -\frac{e^{-y_0}}{\sqrt{y_0}}\Bigr]. \end{aligned}\]Now
\[y = 2\frac{kH}{m}-2\frac{k}{m}\,h, \quad y_0 = 2\frac{kH}{m}-2\frac{k}{m}\,h_0, \quad \sqrt{y},\sqrt{y_0}\sim\sqrt{\tfrac{2kH}{m}}.\]Also
\(\,e^{-y}=e^{-2kH/m}\,e^{2kh/m}\),
\[\,e^{-y_0}=e^{-2kH/m}\,e^{2kh_0/m}.\]Hence
\[t(h)\;\longrightarrow\; \sqrt{\frac{m}{gk}}\, \bigl[e^{2k(h-h_0)/m}-1\bigr].\]In the constant‐density case one finds directly
\[v(h)^2 =\frac{m\,g}{k}\bigl[1-e^{-2k(h_0-h)/m}\bigr], \quad v(h) =\sqrt{\frac{m\,g}{k}}\sqrt{1-e^{-2k(h_0-h)/m}}.\]Finally, solving \(m\,dv/dt=m\,g-k\,v^2\) by separation of variables gives
\[\boxed{ v(t) = \sqrt{\frac{m\,g}{k}}\;\tanh\!\Bigl(t\sqrt{\tfrac{g\,k}{m}}\Bigr), \qquad v_{\rm terminal} = \sqrt{\frac{m\,g}{k}}. }\]Thus the exponential‐atmosphere formula smoothly reduces to the constant-density “tanh” law as \(H\to\infty\).
Exercises for the reader
- Why is the ODE separable (University Year 1)
- Develop the theory if the air resistance is linearly proportional to velocity. (Difficulty: University Year 1-2)
- What are the terminal velocies for the plane and the human if there is no air resistance? Why? (Difficulty: HKDSE F4)
